
Pictured here is a a spinning top. If you throw the top out and simultaneously pull on the string, the top spins. And you get to see this top spend a lot of time balancing on the sharp tip. (I have taken the liberty of choosing a traditional spinning top from my region of the world, instead of the ones you find elsewhere.)
When I was young, we would play with these tops. They were heavy, but the heavier ones where more fun. No, I am not an old fogey; computers and the Internet simply came to us a bit later, and the computers were so slow back then that we would play with real stuff.
Why am I bringing these tops to your attention?

It turns out that the mathematical solution to how a top spins, especially when it is slowing down and starts wobbling, is a particularly important physical example that explains precession and nutation motions. In fact, this is an example which you can find in many classical mechanics textbooks. Moreover, if you solve this problem in high detail, you’ll notice that the Earth’s motion is like a spinning top.
In this understanding, the spinning top exhibits the usual rotation. When it wobbles, you start seeing how precession and nutation come into play (when we speak of the “precession of the equinox” for the Earth’s motion, this is where the name comes from).
But why does a spinning top display this motion?
Yes, if you skip the equations a bit, we can get by with a borderline pass (the next two paragraphs, that is).
I hope that you still remember Newton’s 2nd Law, which simplifies to F = ma in many treatments. Using this law, which is strictly for motion in a line, we can derive what would happen for rotation. We can do this in two ways—either we hand wave and just cross product both sides with position, or we can really be detailed and check what every little bit of material is going to do and integrate over.
In the end, we find that the familiar equations of linear motion is translated, symbol for symbol, to get the equations of rotational motion. For example, the equation F = ma above turns into τ = I · α. In this case, τ (tau) is the torque, I is the moment of inertia that behaves just like the mass (but this time, the different directions get different moments of inertia), and α (alpha) is the angle θ (theta) differentiated twice with time (angular acceleration).
The energy and momentum equations are also translated and conserved, such that, if ω (omega) is the angular speed (rate of rotation), the angular momentum L would be L = I · ω. To know that this is conserved is enough to understand the motion of a wobbling spinning top!
When the top is initially spinning fast, it is pointing straight up and rotating around that axis. Its angular momentum is also pointing straight up and, through dissipative forces, it is decreasing a bit, but still pointing straight up. As it slows down even more, however, the spinning motion starts to fail to counteract the slight and random tilting forces. So, it wobbles a bit. Here, the interesting thing happens.
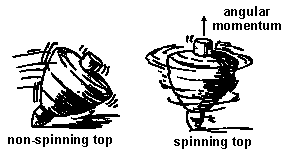
It is tilting a bit, so the main rotation is now giving an angular momentum pointing in some other direction than straight up. But the initial angular momentum is pointing straight up, and this is conserved! So, what is going to happen is that there is some new rotation in the other two possible directions, just enough to vectorial add back to an angular momentum bivector that points straight up. Work out the maths, and this is exactly what explains the precession and nutation motions. Of course, this is quite strenuous, but this explanation is pretty beautiful, especially compared to the other treatments.
Spinning bodies do a lot of things that defy intuition. Back in the day, when we were still playing with these, the scientific community would routinely get letters claiming a way to use spinning bodies to create anti-gravity machines. Understanding their motion is quite amusing just in their own right. We do not need those letters back, but we do lament the loss of childhood for the current generations.
Sources and further reading:
- This treatment comes directly from Landau and Lifshitz Course on Theoretical Physics, Volume 1: Classical Mechanics, which is a very small book. The entire course is particularly painful, but this book is especially easy and nice, probably because classical mechanics is such a well-known subject that Landau had no trouble forwarding good books for their students to do (Landau tends to seek alternative things to talk about, and they must have found nothing to talk about here).
- Feynman also had something to say about this problem in his Lectures Vol 1, but the treatment is a lot more orthodox, and he did not bother finishing up on it.
- For a very complete and thorough treatment of this problem and the link to the Earth’s motion, Goldstein is the currently most used textbook on classical mechanics, which is aimed at those seeking in-depth treatments. Otherwise, other textbooks that deal with it will also be treating it, but not as thoroughly.