Everything You Need to Know About Black Holes

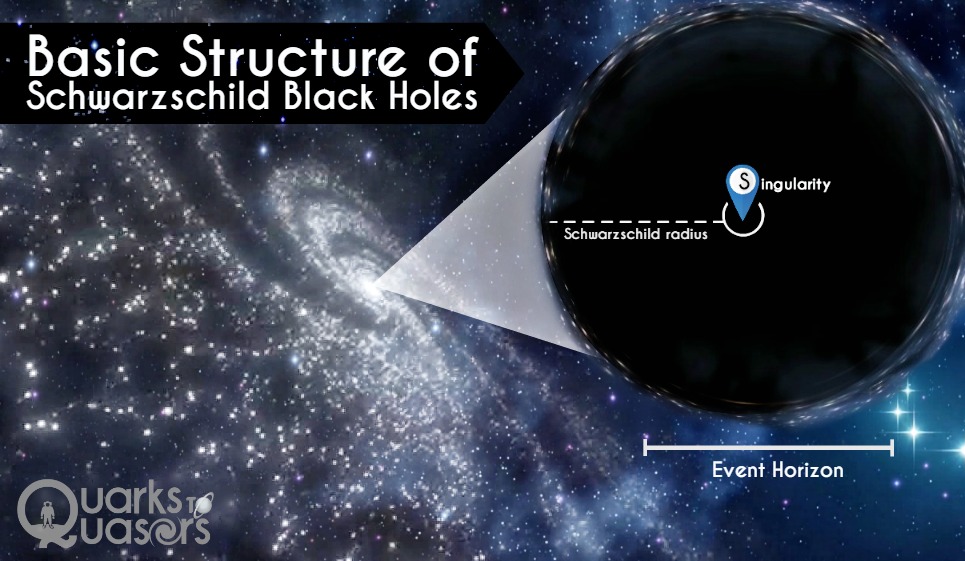
The concept of a “black hole” is one of the most curious in astrophysics. It’s the answer to the question: “What happens if the density of matter in a region becomes so high that not even light can escape?”

The reason this question even arose dates back to Einstein’s prediction of 1916, in his Foundations of General Relativity, that light will be bent in the direction of any nearby mass.

That prediction has been spectacularly confirmed in recent years by the discovery of “gravitational lenses”—where a background source and a foreground mass are so closely aligned that the light from the background source is highly distorted, even to the point of forming an almost complete arc around the foreground mass.

Before 1916, we had not considered that this might be possible. After all, why should photons, the mass-less particles of light, feel the influence of any mass? The surprising answer is that the basic shape of our universe is influenced by each mass within it, like the dimples in a rubber sheet caused by an occasional marble.

Passing light can be thought of as confined to the rubber sheet, and when it passes near a marble it will be deflected from its original path by passing through the dimple. If the dimple becomes too deep, light that passes sufficiently close will be deflected into a spiral path that ends on the marble and it will not escape at all.

The boundary between light paths that just manage to pass a density concentration and those that do not is called the “event horizon” —the greater the mass of a density concentration, the larger the size of this “surface of no return.”

For an object as massive as our Sun, the event horizon is about 6 km in diameter. The matter density needed to form such a black hole is extremely high—about 2 x 10¹⁹ kg per cubic meter. That’s more extreme than the density of an atomic nucleus.

The densest form of matter so far observed in nature is that encountered in so-called neutron stars, an entire star composed only of nucleonic matter. Yet even a neutron star is not dense enough, by a about a factor of 50, to form a black hole of the Sun’s mass.

Density

Curiously, the density needed to form a black hole scales as the inverse square of the total mass. So, the density of a neutron star would be sufficient to form a black hole if the object had about 2,500 times the mass of the Sun. What exactly happens when so much mass is concentrated in the same place is not understood.

Will it compress into some new state, such as the quarks that are thought to be the building blocks of neutrons, or some even more fundamental building block? We just don’t know.

The average density of the Sun—about 1 gram per cubic centimeter—which also happens to be the density of liquid water on Earth’s surface, would be sufficient to form a black hole were it associated with a mass of 100 million times that of the Sun.

This is the mass of the compact objects within the centers of massive galaxies. Continuing this line of reasoning, we can ask: “Do we live in a black hole?”—a question that’s dominated cosmology for the past century.

Is the average density of the universe so high that light and everything else around us could never escape? The answer appears to be “no.” The universe is undergoing an accelerating rate of expansion that implies an insufficient matter density to represent a black hole.

How Do We Know Black Holes Exist?

What evidence is there that the black hole phenomenon actually occurs? At this time, it’s all indirect.

On the scale of individual stars, there are cases where a normal star appears to orbit a compact object that’s very faint but is perhaps ten times as massive as the Sun. Since there are no current theories to explain this, such objects have been called “black hole candidates.”

On larger scales, there’s evidence of a massive but compact object at the center of many, and possibly all, galaxies. The object that resides in the very center of our Milky Way has been studied by tracking the movements of many nearby stars.

The orbits of these stars have been used to deduce that the central object must be about 4 million times more massive than the Sun and that it must be smaller than about 1/1,000 of a light-year (a light-year being equal to approximately 10 trillion kilometers).

Although this is the best current evidence for a very massive, very compact object, it’s important to note that this limit on matter density, of about 10 grams per cubic meter, is still 100 million times smaller than what’s needed—about 1 kg per cubic centimeter—to qualify as a black hole of this mass.

Supermassive Black Holes

It’s thought so-called supermassive black holes—as much as 1 billion times the mass of the Sun—reside in the center of galaxies that are significantly more massive than the Milky Way, particularly those which have an elliptical rather than a disk-like distribution of their stars. Many of these massive galaxies have been host to the “quasar phenomenon” at some point in their history.

The quasar phenomenon is the most energetic type of event that has yet been witnessed in the universe, outshining all of the stars in the hosting galaxies for millions of years, and is understood as a consequence of matter falling into a central massive object.

The extremely strong gravitational attraction of compact massive objects tends to tear apart and pull in anything that comes too close. The tearing action is due to “tidal forces,” the fact that a gravitational force acting on the nearest portions of an object is significantly stronger than that acting on the most distant portions.

This same phenomenon causes tides on Earth, since the gravitational attraction of the Moon is significantly greater on the side of Earth facing the Moon than the side facing away. As material is pulled toward the compact massive object it tends to gather in what is called an “accretion disk,” a very hot, rapidly rotating structure that channels material toward the central object.

The reason for the rapid spin is the preservation of angular momentum, akin to what a slowly rotating ice skater experiences when they draw in their arms from a more extended position. Any small initial rotational motion is strongly amplified during contraction.

The high temperature is the result of the high-speed collisions between material falling in and that already in place.

The final stage of channelling material inward is a challenging one, since somehow the energy associated with the rapid rotation must first be shed. The solution nature has found to this problem is dramatic: the rotational energy of the accretion disk is shed by sending high-speed jets of matter out along the rotation axis of the disk.

The same jet ejection phenomenon is found to apply over an extremely wide range of scales, from the formation process of individual stars, such as the Sun, to that of quasar accretion disks, where the result can be the largest distinct objects yet seen, measuring millions of light years from end to end.

But are the compact massive objects seen on a wide range of scales truly black holes, in the sense of having achieved a sufficient mass density to become disconnected from the rest of the universe? Or are they merely a highly condensed state of matter that we don’t yet understand?

Seeing is Believing

Direct evidence for the black hole phenomenon might be possible if an image could be made of the event horizon. While this has not yet been done, the best prospects might come from looking at the object at the center of our own galaxy, since it provides the best combination of a large event horizon size with the closest possible distance.

The expected image size is about 0.2 milli-arcseconds, or about 10,000 times smaller than the typical image size of a star observed with an optical telescope from the ground.

The required resolution could be achieved by a network of radio telescopes separated by thousands of kilometers and observing at wavelengths of about a millimeter. Such a project—the Event Horizon Telescope (EHT)—is being developed by astronomers at the Smithsonian Institution. If successful, the EHT will demonstrate that nature has truly found a way to squeeze matter together to a required density that even light cannot escape.

Probing the detailed shape of the event horizon and how it depends on total mass should provide clues about the state of matter under these extreme circumstances; circumstances that we can not approximate in a laboratory.

Its likely that nature will have some surprises waiting for us when we do. Each time we peel off a layer of the onion there seems to be another one inside.

See more Explainer articles on The Conversation. Robert Braun is Chief Scientist for CASS at CSIRO.