Why Do Rivers Curve The Same?

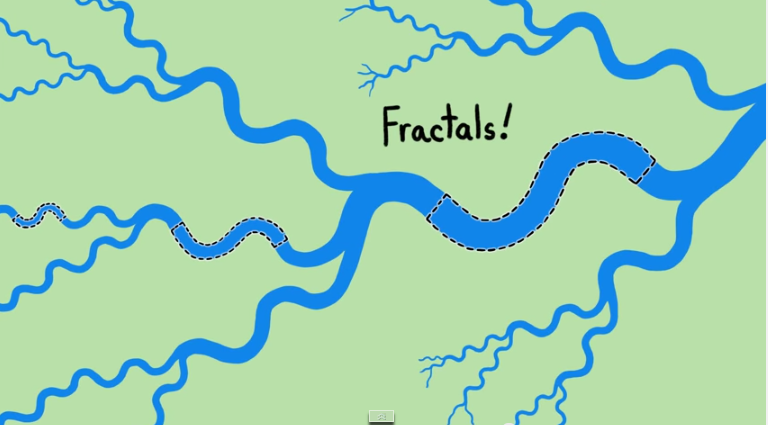
Here's a fun fact that you might not have known: All over the world, rivers generally follow the same pattern. And no, we don't mean that they follow the same trend of flowing down stream. They follow the same pattern. Ultimately, the length of one of the familiar S-shaped curves that we see in rivers is around six times the width of the channel. And we find this time and time again.

So not only are many rivers strikingly similar, this means that rivers are kind of like fractals. In mathematical terms, fractals are geometric figures that typically display self-similar patterns. The term “self-similarity” can be most readily understood by thinking of a camera that zooms in on an image. Usually when you zoom in on a photograph, you see finer points, different details, and new structures. Not so with fractals. When you zoom in on a true fractal, no new detail appears. Nothing changes. Rather, the same exact pattern repeats over, and over.

Of course, rivers are not perfect fractals. However, the tiny, bendy streams are just smaller versions of their bigger relatives. We see this pattern over and over, find out why in the video below.

WATCH: Why Rivers Curve